Burhan Al-Tatbiq: Between the Philosophers and Cantor

Burhan Al-Tatbiq: Between the Philosophers and Cantor

Author: Karkooshy  | Original Source

﷽

In arguing for the impossibility of an infinitely large body, the Philosophers utilized what is now referred to as “Burhan al-Tatbiq” ('Proof from One-to-One Correspondence'). The proof is built upon the fact that adding or subtracting a quantity from any amount, cannot net that same amount ^[1]. So if we supposed the existence of an infinitely long body, and then we supposed the removal of a finite part from this length ^[2], then what remains after the removal would also be infinite. This conflicts with what was already established (namely, that subtracting a quantity from any amount, cannot net that same amount), since the length would be infinite both before and after the subtraction. Thus, the first supposition (i.e. that an infinite body exists) is an impossibility.

Burhan Al-Tatbiq and the Philosophers

In developing the above argument in more detail, Ibn Sina writes^[3]:

 لا يتأتى أن يوجد مقدار ذو وضع غير متناه لأنه إما أن يكون غير متناه من الأطراف كلها أو غير متناه من طرف

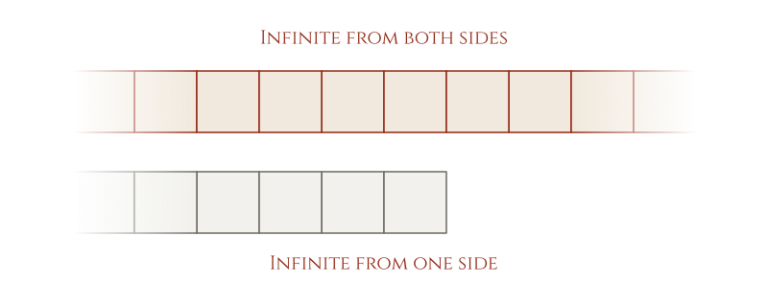
It is impossible for there to exist an infinite length, because either this length would be infinite from all sides, or only infinite from one side.

 

فإن كان غير متناه من طرف أمكن أن يفصل منه من الطرف المتناهي جزء بالتوهم فيؤخذ ذلك المقدار مع ذلك الجزء على حدة وبانفراده شيئاً على حدة

If its infinitude were from only one side, then it would be possible for us to take the endpoint, and imagine the removal of a [finite] length from it. Treat the amount before the removal as its own length, and the net amount after the removal as another.

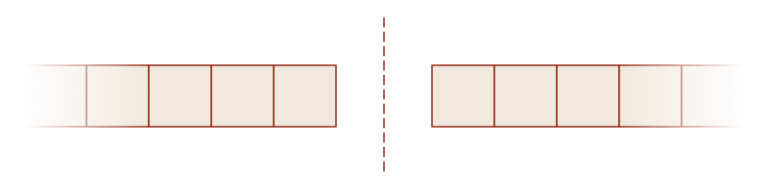
 ثم نطبق بين الطرفين المتناهيين في التوهم فلا يخلوا إما أن يكونا بحيث يمتدان معاً متطابقين في الإمتداد فيكون الزائد والناقص متساويان – وهذا محال

Then we correspond the two lengths with each other starting from the endpoints. Now, either both lengths extend to infinity together [such that there is a one-to-one correspondence between all their parts]. But in this case, the lesser length would be equal to the greater one – and that is impossible.

  

وإما أن يمتد بل يقتصر عنه فيكون متناهياً والفصل أيضاً كان متناهياً فيكون المجموع متناهياً فالكل متناه

Or, the lesser one extends with the other for some of its length, but the correspondence eventually comes to an end [such that there is a one-to-one correspondence between only a part of the greater length, with the entirety of the lesser one]. In this case, the lesser length would be finite, the difference between the two is finite, so the total [i.e. the greater length] would be finite as well. Entailing that both lengths be finite [since the sum of two finites, is finite].

 وإما إذا كان غير متناه من جميع الأطراف فلا يبعدان يفرض فيه مقطع تتلاقى عليه الأجزاء ويكون طرفاً ونهاية ويكون الكلام في الأجزاء أو الجزئين كالكلام في الأول وبهذا يتأتى البرهان على أن العدد المترتب الذات الموجود بالفعل متناه

And if the supposed length were infinite from all sides, it would not be impossible to cut this infinite length to create an end point for each of its parts, and the previous argument would then apply to each and all of those parts. And from this it is established that a sequential and actually existent amount, must be finite.

 

However, since the Philosophers believed in the infinitude of the events preceding the present moment, and to avoid the aforementioned proof backfiring on them, they maintained that the proof only works on amounts whose parts all exist simultaneously. For example, the parts of a body, which is why it is impossible for an infinitely large body to exist. As for the events of the past, they do not concurrently exist, and so the Philosophers claimed that Burhan al-Tatbiq does not apply there.

In response, the Mutakalimun argued that this condition (i.e. that Burhan al-Tatbiq will only apply if the parts of the amount in question existed simultaneously) is completely ad hoc^[4]. Rather, Burhan al-Tatbiq will work on any actual infinite^[5] that accepts increases or decreases. This is because the proof is based on the fact that the amount after the increase or decrease, cannot equal the amount before the increase or the decrease, whereas this would be the case if the initial amount were infinite. And since the Philosophers believed that the number of past events is an actual infinite, and that this number increases as time progresses^[6], then Burhan al-Tatbiq applies to the sequence of past events as well.

Burhan Al-Tatbiq and Cantor’s Transfinite Set Theory

Some today have attempted to use Cantor’s transfinite set theory to respond to Burhan Al-Tatbiq. They argue that there actually are infinite amounts that are greater or less than other infinite amounts, and so Burhan Al-Tatbiq does not disprove what both the Philosophers and the Mutakalimun claim it does^[7].

For example: they claim that there are an infinite number of real numbers, and that there are an infinite number of natural numbers. There are however, an infinite number of reals between any two reals. On the other hand, there are only a finite number of naturals between any two naturals. And so there cannot be a one-to-one correspondence between the elements of the two sets. Thus, the set of all reals is greater than the set of all naturals, even though both are infinite.

This contention however is fundamentally based on ambiguous language, and it quickly falls apart when we examine the meanings used more carefully. In particular we are interested in what the opponent means when they say things like “number”, “set”, and “there are an infinite number of real numbers” or “there are an infinite number of rationals”.

Numbers

The word “number” is used in two senses:

1. First: as a count of the parts in an extra-mental amount. For example: when one sees Zayd, and ‘Amr, one knows that they are collectively two humans. In this sense, the extra-mental existents are Zayd and ‘Amr. “Two” is a mental abstraction that the mind uses to describe the size of this group. We call this an extracted abstraction (I’tibar Intiza’i), because it is one that was extracted from extra-mental reality.
2. Second: as a mental concept without reference to any extra-mental amount. For example: when we mentally recite natural numbers without using them as a count for anything. So we mentally recite to ourselves: one, two, three, four, five…etc. In this sense, there are only the numbers that exist in the mind of the reciter, nothing extra-mentally. We call any one of those numbers an invented abstraction (I’tibar Ikhtira’i), because the mind of the reciter is inventing those concepts.

We argue that there can never exist an infinity of numbers, in either sense.

As for the first sense, a number can only be abstracted by counting the parts of an amount. For the opponent to claim that there are an infinity of numbers therefore, is tantamount to claiming that they finished counting an infinite number of parts. This is patently impossible however, since an infinite amount would be endless by definition, and so one would never finish counting it. At any given step of this counting process, the number of counted parts will always be finite, and this counting process should never come to an end^[8].

As for the second sense, for the opponent to claim that there are an infinity of numbers, is tantamount to claiming that they finished inventing an infinite number of concepts in their mind. This too is impossible, because each invention is a task, and an infinite sequence of tasks cannot be finished. Since finishing implies coming to an end, infinite implies endlessness, and what is endless cannot come to an end.

And if the opponent argues: “when we say there are an infinity of numbers, we are not using ‘infinity’ as a count of actualized extra-mental existents, nor are we using it as a count of invented abstractions in the mind. We are simply supposing the existence of an infinite amount of numbers, and we’re performing mathematical operations on this supposed infinity.”

We respond: we do not dispute that it is possible to suppose the existence of an actual infinity of numbers. But it is possible to suppose impossibilities. As such, merely supposing the unsoundness of Burhan Al-Tatbiq (which is what you’re doing by supposing the existence of an actual infinity of numbers) does not suffice as a response to the proof. And we have already demonstrated the impossibility of your supposition.

Sets

A set is a collection of discrete objects. When the opponent argues using Cantor’s theories however, they’re referring to sets of numbers (e.g. “the set of all real numbers” or “the set of all naturals numbers”). “Number” here is being used in the second sense outlined above. 

The opponent’s mistake is treating the set as an entity that pre-exists his conception of it. Rather, sets are mental constructions that do not exist independently from the mind.

We argue that a set with an infinite cardinality cannot exist. This is for two reasons:

1. First: for a set to exist it must be constructed, and it is impossible to finish constructing an infinite set, because an infinite sequence of tasks cannot be finished.
2. Second: a set with an infinite cardinality would be one that is comprised of an infinity of numbers. And we have already shown that it is impossible for an infinity of numbers to exist, so a set with an infinite cardinality cannot exist.

Infinitude of Numbers

If there cannot exist an infinity of numbers, in what sense are they “infinite”? The answer is that the infinitude of numbers is only a potentiality. In other words, the amount of actualized numbers is necessarily finite, but there is never a point when it would be impossible to invent a number that is greater or less than one already in existence.

The above also applies to the cardinality of sets. At any point in time, the number of elements in a given set is necessarily finite. However, there is never a point when it would be impossible to construct a set that is larger than the one given.

So since Cantor’s transfinite set theory supposes the existence of infinite cardinalities, and given the fact that this supposition is actually an impossibility, the opponent’s objection to Burhan al-Tatbiq falls.


Footnotes

[1] If the alternative were possible, then it would be possible for a lesser amount to equal a greater one. This is impossible, because that would make the lesser amount not lesser, and the greater amount not greater.


[2] We would not be supposing an impossibility in this case, since each part of any body exists contingently, and contingents accept non-existence.


[3] Al-Najatu fi Al-Mantiqi wa Al-Ilahiyat (pg 71).


[4] We can also demonstrate that, according to the principles of the Philosophers, assuming an infinite past will entail it being possible for an infinite amount of concurrently existent parts to exist. And since the Philosophers accept that the latter is impossible, then they must accept that the former is so as well.

This is given that they believe in the existence of beginningless bodies, and that matter is infinitely divisible. So we say: suppose that those beginningless bodies were continuously dividing into two by breaking apart since eternity past, such that the number of bodies at any given moment, is double the number of bodies that existed in the previous moment. And because this would have been occurring since eternity past, the numbers of bodies at any given moment must be an actual infinite amount of concurrently existent parts.


[5] Actual Infinite: an amount whose actualized parts total infinity. “Actualized” meaning: entered into existence.

For example: if someone were to claim that they finished counting all the natural numbers, then they’re claiming that the amount of counted numbers is an actual infinite.

Contrasted with a potential infinite, which is a finite amount that accepts continuous increases. One that never reaches a point when it becomes impossible for it to increase. One that never reaches a point when the number of actualized parts totals infinity.<
For example: the count of natural numbers. This is because we can name a greater natural for any natural that is given to us. The amount of counted numbers is always finite, but there is no point when it would be impossible to name a greater natural than the one given.


[6] The number of events that entered into existence before today, is greater than the number of events that entered into existence before last year. Since the number of events that entered into existence before today, is equal to the number of events that entered into existence before last year, plus the number of events that entered into existence between today and last year. Thus, the number of actualized events increases as time progresses.


[7] Since Burhan Al-Tatbiq depends on the fact that an infinite amount cannot be greater or less than another infinite amount. And so, any amount which accepts increases or decreases cannot be infinite.


[8] And so by way of mere counting, the counter can never know whether what he is counting is an actual infinite, or if it is some great finite amount that he simply has not finished counting yet.